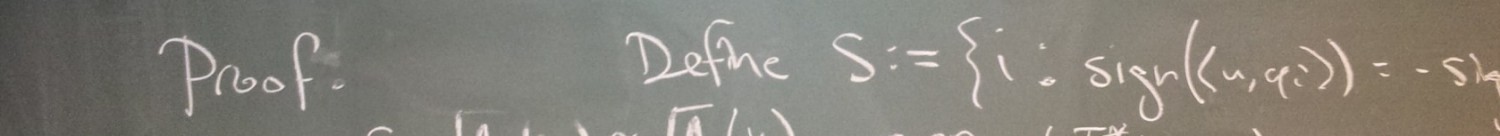Math exposition on YouTube

This summer, 3Blue1Brown launched the third installment of the Summer of Math Exposition contest. Every year, I’ve been impressed and inspired by the quality of some of the videos in this contest. Over the last couple of months, I worked with Boris Alexeev and John Jasper to throw our hat in the ring:

I was surprised by how much I learned from this experience. In what follows, I reflect on the process of making this video.

3b1b podcast #3

Grant Sanderson (of 3Blue1Brown fame) recently launched the 3b1b podcast as part of the SoME1 math exposition challenge. This page annotates the third episode of the podcast:

In this episode, Grant Sanderson (GS) interviews Steven Strogatz (SS) about research, pedagogy, and exposition, and what follows is some commentary by John Jasper (JJ), Emily King (EK), Clayton Shonkwiler (CS), and me (DM). Feel free to discuss further in the comments.

3b1b podcast #1

Grant Sanderson (of 3Blue1Brown fame) recently launched the 3b1b podcast as part of the SoME1 math exposition challenge. To celebrate, some friends and I decided to launch an experiment on this blog by annotating the first episode of the podcast:

In this episode, Grant Sanderson (GS) interviews Alex Kantorovich (AK) about all things academia, and what follows is some commentary by John Jasper (JJ), Hans Parshall (HP), and me (DM). Feel free to discuss further in the comments.

Polymath16, seventeenth thread: Declaring victory

This is the seventeenth “research” thread of the Polymath16 project to make progress on the Hadwiger–Nelson problem, continuing this post. This project is a follow-up to Aubrey de Grey’s breakthrough result that the chromatic number of the plane is at least 5. Discussion of the project of a non-research nature should continue in the Polymath proposal page. We will summarize progress on the Polymath wiki page.

At this point, we are finalizing a draft of a paper by D.H.J. Polymath. Like Aubrey’s original paper, this will be submitted for publication in Geombinatorics.

This post concludes the Polymath16 project. Of course, we anticipate that folks will continue to make progress on this problem on their own. If you’d like to update the Polymath16 community about your progress, feel free to comment on this post.

I asked Aubrey to offer a few words to reflect on our project:

Codes and Expansions (CodEx) Seminar

Last summer, I launched an online seminar with Joey Iverson, John Jasper, and Emily King on the theory and applications of harmonic analysis, combinatorics, and algebra. We meet on Tuesdays at 1pm (Eastern time).

We’re kicking off the spring semester on January 26 with a talk from Steve Flammia on recent progress on Zauner’s conjecture.

Click here for the Zoom link and to sign up for the mailing list.

Click here for a list of past and upcoming talks.

Click here for the CodEx YouTube channel.

A graph coloring problem from quantum physics (with prizes!)

What follows is a nice problem that was recently posed by Mario Krenn. Progress on this problem may lead to new insights in quantum physics (see this, this, this, and this for details). In addition, Mario has announced two cash prizes to encourage work in this direction. (!)

Consider a weighted and edge-colored bipartite graph consisting of

• disjoint vertex sets and ,
• an edge set ,
• an edge coloring , and
• a complex vertex weighting .

MATH 2568: Linear Algebra

This spring, I’m teaching a first-semester course on Linear Algebra at the Ohio State University.

Click here for a draft of my lecture notes.

I will update the above link periodically. Feel free to comment below.

Update #1: Added two sections to Chapter 1.

Update #2: Updated Chapter 1 and started Chapter 2.

Update #3: Added a section to Chapter 2.

Update #4: Added another section to Chapter 2.

Update #5: Added another section to Chapter 2.

Update #6: Added Chapter 3 and started Chapter 4.

Update #7: Updated Section 4.1.

Update #8: Added Section 4.2.

Update #9: Started Section 4.3.

Update #10: Added Section 4.4.

Kopp’s Whisky Prize

At the end of his recent CodEx talk, Gene Kopp posed a problem with a prize attached to it. I was excited to learn about this, so I enlisted both Gene Kopp and Mark Magsino to help me write this blog entry to provide additional details.

First, let denote the set of matrices in such that

Here, denotes conjugate transpose, denotes entrywise squared modulus, denotes the identity matrix, and denotes the all-ones matrix. In words, the columns of form an equiangular tight frame (ETF) for of size .

Polymath16, sixteenth thread: Writing the paper and chasing down loose ends, II

This is the sixteenth “research” thread of the Polymath16 project to make progress on the Hadwiger–Nelson problem, continuing this post. This project is a follow-up to Aubrey de Grey’s breakthrough result that the chromatic number of the plane is at least 5. Discussion of the project of a non-research nature should continue in the Polymath proposal page. We will summarize progress on the Polymath wiki page.

The paper writing has found a second wind between Philip Gibbs, Aubrey de Grey, Jaan Parts and Tom Sirgedas. For reference, I wanted to compile a list of related publications that have emerged since starting our project. (Feel free to any references I missed in the comments.) There has certainly been a bit of activity since Aubrey’s paper first hit the arXiv two years ago!

P. Ágoston, Probabilistic formulation of the Hadwiger–Nelson problem.

F. Bock, Epsilon-colorings of strips, Acta Math. Univ. Comenianae (2019) 88: 469-473.

G. Exoo, D. Ismailescu, A 6-chromatic two-distance graph in the plane, arXiv preprint arXiv:1909.13177 (2019).

G. Exoo, D. Ismailescu, The chromatic number of the plane is at least 5: A new proof, Discrete & Computational Geometry (2019): 1-11.

G. Exoo, D. Ismailescu, The Hadwiger-Nelson problem with two forbidden distances, arXiv preprint arXiv:1805.06055 (2018).

N. Frankl, T. Hubai, D. Pálvölgyi, Almost-monochromatic sets and the chromatic number of the plane, arXiv preprint arXiv:1912.02604 (2019).

M. J. H. Heule, Computing a Smaller Unit-Distance Graph with Chromatic Number 5 via Proof Trimming, arXiv preprint arXiv:1907.00929 (2019).

M. J. H. Heule, Searching for a Unit-Distance Graph with Chromatic Number 6, SAT COMPETITION 2018: 66.

M. J. H. Heule, Trimming Graphs Using Clausal Proof Optimization, In International Conference on Principles and Practice of Constraint Programming, pp. 251-267. Springer, Cham, 2019.

J. Parts. A small 6-chromatic two-distance graph in the plane, Geombinatorics, vol. 29, No. 3 (2020), pp.111-115.

J. Parts. Graph minimization, focusing on the example of 5-chromatic unit-distance graphs in the plane, Geombinatorics, vol. 29, No. 4 (2020), pp.137-166.

Polymath16, fifteenth thread: Writing the paper and chasing down loose ends

This is the fifteenth “research” thread of the Polymath16 project to make progress on the Hadwiger–Nelson problem, continuing this post. This project is a follow-up to Aubrey de Grey’s breakthrough result that the chromatic number of the plane is at least 5. Discussion of the project of a non-research nature should continue in the Polymath proposal page. We will summarize progress on the Polymath wiki page.

At this point, much of the effort is transitioning to the writing stage, which is taking place on Overleaf. See this comment to obtain writing privileges for the paper. This thread can be used to discuss the write-up as well as any remaining research items.