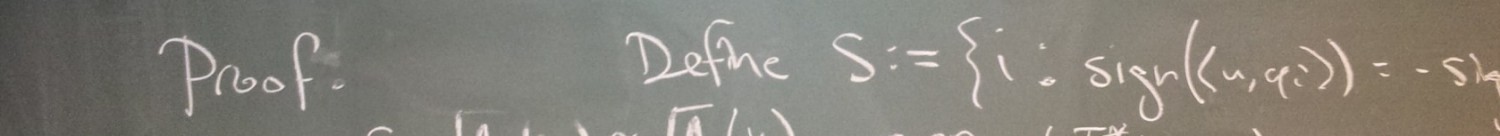Kopp’s Whisky Prize

At the end of his recent CodEx talk, Gene Kopp posed a problem with a prize attached to it. I was excited to learn about this, so I enlisted both Gene Kopp and Mark Magsino to help me write this blog entry to provide additional details.

First, let denote the set of matrices in such that

Here, denotes conjugate transpose, denotes entrywise squared modulus, denotes the identity matrix, and denotes the all-ones matrix. In words, the columns of form an equiangular tight frame (ETF) for of size .

One particularly interesting family of ETFs are of the form . In the quantum information theory community, these are known as symmetric, informationally complete positive operator–valued measures; the modern abbreviation for this mouthful is SIC. In his PhD thesis, Zauner predicted that SICs exist for every , but to date, they are only known to exist for finitely many . Almost all existing SICs are constructed by spinning a seed vector with a representation of the Weyl–Heisenberg group, and the entries of the seed vector are expressible in radicals. Frustratingly, the complexity of this description appears to grow like , which suggests that this is not the best representation for these seed vectors.

In 2018, Gene discovered an alternative that seems to be the “correct” representation. Specifically, the squares of the entries of appear to be obtained by applying a Galois automorphism to the Stark units of an abelian extension of with ramification at the primes dividing and at one infinite place. In the case when is prime, Gene gives a precise recipe to construct the entries of from their squares.

These equiangular tight frames exhibit field structure that is completely different from other known constructions. In general, given , the triple products with form an invariant over the left-action of and the right-action of . For all known ETF constructions other than SICs, either the ETF belongs to a continuum of inequivalent ETFs, or the triple products all belong to a cyclotomic field. This observation compelled Gene to formulate the following problem:

Kopp’s Whisky Prize. Find and such that

(i) ,

(ii) is an isolated point in , and

(iii) there exists such that .

To clarify, (i) prevents from being (the Naimark complement of) a SIC, (ii) prevents from belonging to a continuum of inequivalent ETFs, and (iii) prevents from having all triple products belonging to a cyclotomic field. (The field is the union of all cyclotomic fields.) Given a candidate solution , then (i) is easy to certify and (iii) reduces to a straightforward MAGMA query, while (ii) requires a bit more effort. One way to certify (ii) is to estimate the rank of the Jacobian matrix of the defining equations at . Complexifying these equations produces an algebraic variety of “quasi-ETFs.” If an ETF is an isolated point in , then it is also an isolated point in . For reference, Gene implemented this idea in a Mathematica notebook to certify that a particular member of satisfies (ii).

Finally, why is it called a whisky prize? The first person to discover any such and send it to Gene will be rewarded with a bottle of Dalwhinnie Winter’s Frost Single Malt Scotch Whisky, House Stark Game of Thrones Limited Edition. Mind the double-pun/homage: (House Stark Stark units) and (Game of Thrones Game of Sloanes).

Send your solution to Gene Kopp at this page.